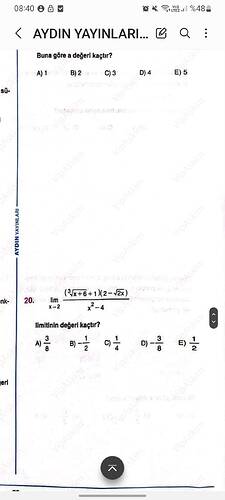
What is the limit of the given expression as x approaches 2?
Answer:
To solve the given limit problem, we have:
\lim_{x \to 2} \frac{(\sqrt[3]{x+6} + 1)(2 - \sqrt{2x})}{x^2 - 4}
Step 1: Simplify the Denominator
The denominator x^2 - 4 can be factored as a difference of squares:
x^2 - 4 = (x - 2)(x + 2)
Step 2: Examine the Numerator
The numerator is (\sqrt[3]{x+6} + 1)(2 - \sqrt{2x}) . To better understand how to simplify it or apply limits, let’s examine both parts separately:
- As x \to 2 , \sqrt[3]{x+6} \to \sqrt[3]{8} = 2 .
- As x \to 2 , \sqrt{2x} \to \sqrt{4} = 2 .
Both these substitutions result initially in:
- \sqrt[3]{x+6} + 1 \to 3
- 2 - \sqrt{2x} \to 0
Therefore, the numerator tends to 3 \cdot 0 = 0 .
Step 3: Factor and Simplify Factors
The numerator, resulting in an initial 0 \cdot 3 = 0 , suggests potential factorization or cancellation with the denominator is needed.
Now, let’s substitute x = 2 + h where h \to 0 :
- \sqrt[3]{2+h+6} = \sqrt[3]{8 + h} \approx 2 + \frac{1}{12}h (using binomial expansion)
- \sqrt{2(2+h)} = \sqrt{4 + 2h} \approx 2 + \frac{1}{2}h
Substitute these into the expansion:
(\sqrt[3]{x+6} + 1)(2-\sqrt{2x}) \approx \left(3 + \frac{1}{12}h\right)\left(-\frac{1}{2}h\right)
Resulting in:
= \left(3 + \frac{1}{12}h\right)\left(-\frac{1}{2}h\right)
= -\frac{3}{2}h - \frac{1}{24}h^2
Step 4: Evaluate the Limit
Now divide this expanded numerator part by the factorized denominator (x-2)(x+2) = h(4 + h).
Thus,
\lim_{h \to 0} \frac{-\frac{3}{2}h - \frac{1}{24}h^2}{h(4+h)}
= \lim_{h \to 0} \frac{h(-\frac{3}{2} - \frac{1}{24}h)}{h(4 + h)}
Now cancel h:
= \lim_{h \to 0} \frac{-\frac{3}{2} - \frac{1}{24}h}{4 + h}
\approx \frac{-\frac{3}{2}}{4} = -\frac{3}{8}
Final Answer:
Hence, the limit is
-\frac{3}{8}